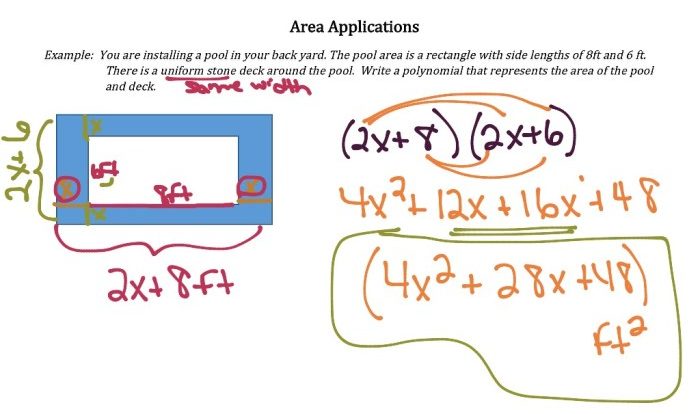
Evaluate the spherical coordinate integral. – Evaluating spherical coordinate integrals is a fundamental technique in mathematics and physics, offering a powerful tool for solving complex problems involving three-dimensional geometry. This guide delves into the intricacies of spherical coordinate integrals, exploring their formulation, integration techniques, and practical applications.
The spherical coordinate system provides a natural framework for describing three-dimensional space, particularly in situations with spherical symmetry. By converting Cartesian coordinates to spherical coordinates, integrals can often be simplified and solved more efficiently.
Coordinate System Conversion: Evaluate The Spherical Coordinate Integral.
The spherical coordinate system is a three-dimensional coordinate system that uses three coordinates to locate a point in space: radial distance (r), polar angle (θ), and azimuthal angle (φ). The radial distance is the distance from the origin to the point, the polar angle is the angle between the positive z-axis and the line connecting the origin to the point, and the azimuthal angle is the angle between the positive x-axis and the projection of the line connecting the origin to the point onto the xy-plane.
To convert between spherical and Cartesian coordinates, the following equations can be used:
- x = r sin θ cos φ
- y = r sin θ sin φ
- z = r cos θ
The spherical coordinate system has several advantages over the Cartesian coordinate system. First, it is often more natural to describe the location of a point in space using spherical coordinates. For example, the location of a point on the surface of a sphere is naturally described using radial distance and polar and azimuthal angles.
Second, the spherical coordinate system is often more convenient to use for certain types of calculations. For example, the volume of a sphere can be calculated more easily using spherical coordinates than using Cartesian coordinates.
However, the spherical coordinate system also has some limitations. First, it can be difficult to visualize the location of a point in space using spherical coordinates. Second, the spherical coordinate system can be more difficult to use for certain types of calculations.
For example, the distance between two points in space is more difficult to calculate using spherical coordinates than using Cartesian coordinates.
Integral Formulation
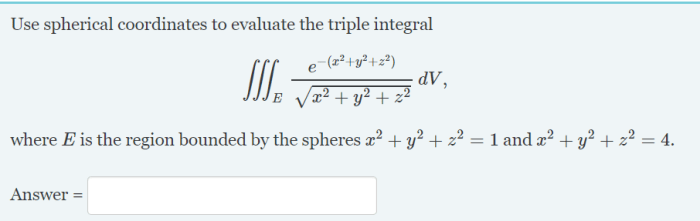
The spherical coordinate integral is an integral that is evaluated over a region in space that is defined using spherical coordinates. The spherical coordinate integral is given by the following equation:
∫∫∫ Vf(r, θ, φ) dV = ∫ 02π∫ 0π∫ 0∞f(r, θ, φ) r 2sin θ dr dθ dφ
where V is the region of integration, f(r, θ, φ) is the integrand, and dV is the volume element in spherical coordinates.
The Jacobian in the integral formulation is the determinant of the transformation matrix that is used to convert between spherical and Cartesian coordinates. The Jacobian is given by the following equation:
J = |∂(x, y, z)/∂(r, θ, φ)| = r 2sin θ
The Jacobian is important because it ensures that the integral is evaluated over the correct region in space.
The spherical coordinate integral can be used to evaluate a variety of integrals. Some examples of integrals that can be evaluated using spherical coordinates include:
- The volume of a sphere
- The surface area of a sphere
- The center of mass of a sphere
- The moment of inertia of a sphere
Integration Techniques

There are a number of techniques that can be used to evaluate spherical coordinate integrals. One common technique is the method of substitution. The method of substitution involves substituting the spherical coordinates into the integrand and then evaluating the integral using the appropriate substitution rules.
Another technique that can be used to evaluate spherical coordinate integrals is the use of spherical symmetry. Spherical symmetry occurs when the integrand is a function of r only. When the integrand is spherically symmetric, the integral can be evaluated by integrating over the radial coordinate only.
A third technique that can be used to evaluate spherical coordinate integrals is the application of integration by parts. Integration by parts can be used to reduce the order of the integral by one. This can make the integral easier to evaluate.
Applications
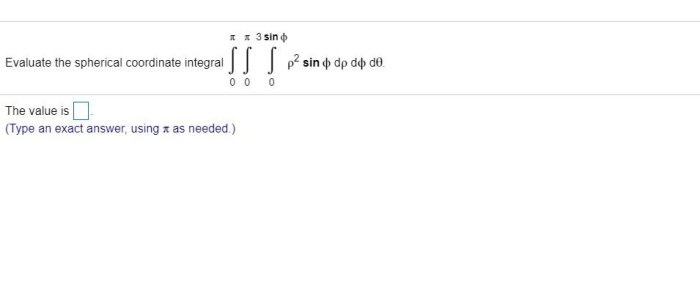
Spherical coordinate integrals are used in a variety of applications in physics, engineering, and mathematics. Some examples of applications where spherical coordinate integrals are used include:
- Calculating the gravitational force between two objects
- Calculating the electric field of a charged sphere
- Calculating the heat transfer from a spherical object
- Calculating the moment of inertia of a rotating object
- Calculating the volume of a region in space
Spherical coordinate integrals are a powerful tool that can be used to solve a variety of problems in physics, engineering, and mathematics.
FAQ Summary
What is the significance of the Jacobian in spherical coordinate integrals?
The Jacobian is a determinant that accounts for the change in volume when transforming from Cartesian to spherical coordinates. It ensures that the integral remains valid under the coordinate transformation.
How can spherical symmetry simplify spherical coordinate integrals?
Spherical symmetry implies that the integrand depends only on the radial distance from the origin. This allows for the separation of variables, leading to simpler integrals that can be evaluated more easily.
What are some practical applications of spherical coordinate integrals?
Spherical coordinate integrals are used in various applications, such as calculating the gravitational field of a spherical mass distribution, determining the volume of a spherical shell, and solving wave equations in spherical coordinates.